48 - Algebra Questions Answers

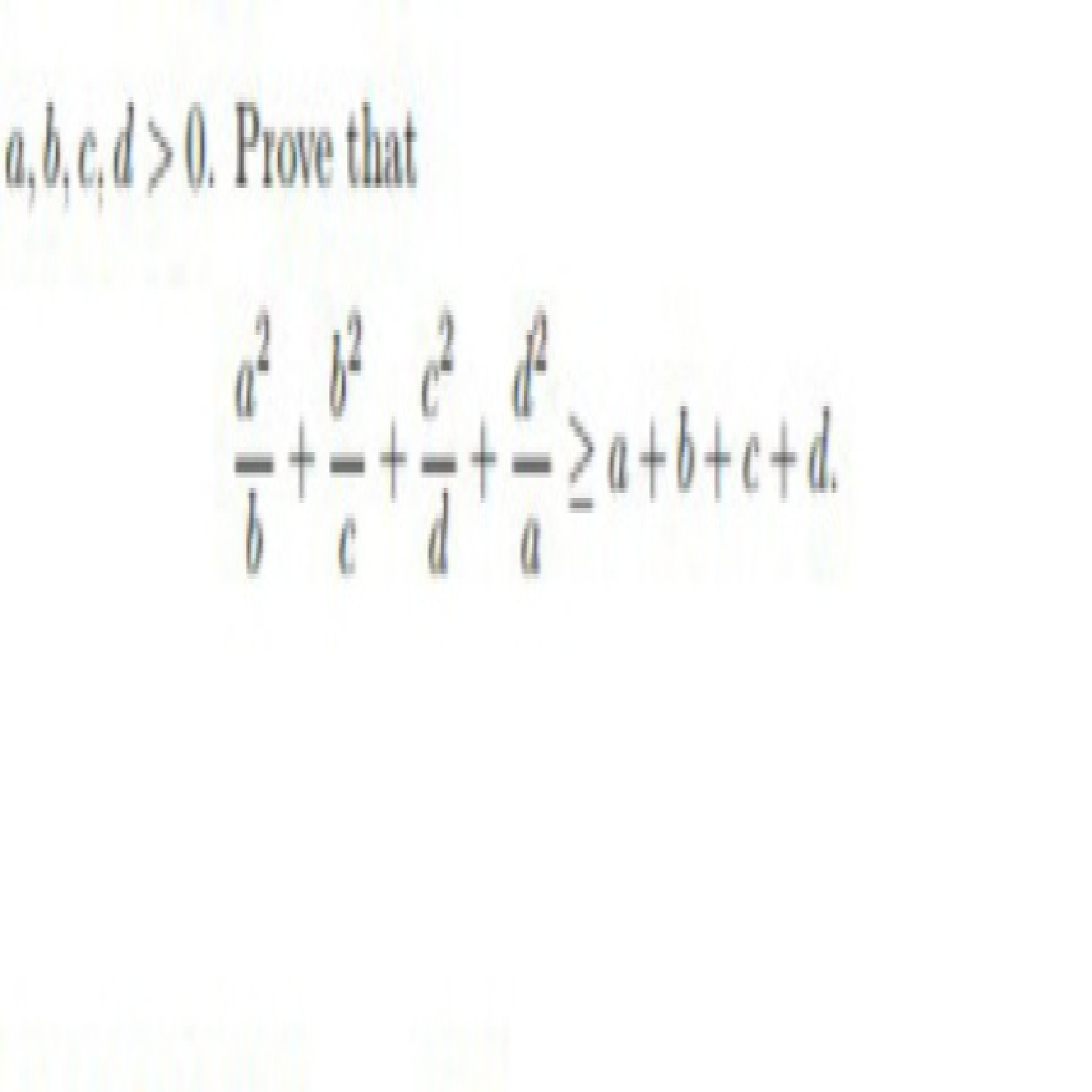
$$\begin{gathered} Probability to get following differences \\ difference probability \\ 0 6/36 \\ 1 10/36 \\ 2 8/36 \\ 3 6/36 \\ 4 4/36 \\ 5 2/36 \\ So required probability =1-2\left[\frac{6}{36}\left(\frac{4}{36}+\frac{2}{36}\right)+\frac{10}{36}\left(\frac{2}{36}\right)\right] \\ now solve \\ problem is based on integral values so area is not very useful \end{gathered}$$

$$\begin{gathered} \frac{x}{1-x^2}=\frac{1}{1-x}-\frac{1}{1-x^2} \\ break all terms similarly and solve \end{gathered}$$

two times sign change so 2 positive roots

if ax²+bx+c is positive for real x

then b²< 4ac similarly others

on adding all inequalities we get b² + c² + a²< 4(ac+ab+bc)

now get answer 

general term of the series = r(n+1-r)² = r(n+1)² + r³ - 2(n+1)r²

now put sigma then solve                                       

  ∑ r ⁿC_rx^ry^n-r

= ∑ r ⁿC_rx^r(1-x)^n-r

= ∑ r n!/r!(n-r)! x^r(1-x)^n-r

= n (n-1)!/(r-1)!(n-r)! x^r(1-x)^n-r 

= n ^(n-1)C_(r-1) x^r(1-x)^n-r

= n ^(n-1)C_r x^r+1(1-x)^n-r-1             let r = r+1

= nx  ^(n-1)C_r x^r(1-x)^n-r-1 

^{now solve} 

exact 0 and lim tends to 0 are different

OPTION

ordered way INOOPT

total words = 6!/2! = 360

so rank = (360/6)*2+([60*2]/5)*3+(24/4)*3+(6/3)*0+(2/2)*1+1 = 120+72+18+0+1+1 = 212