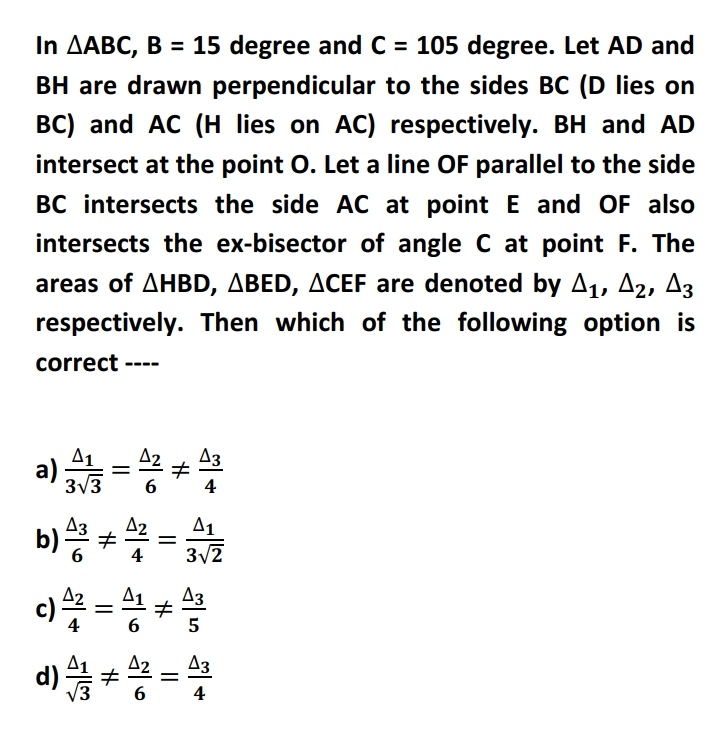
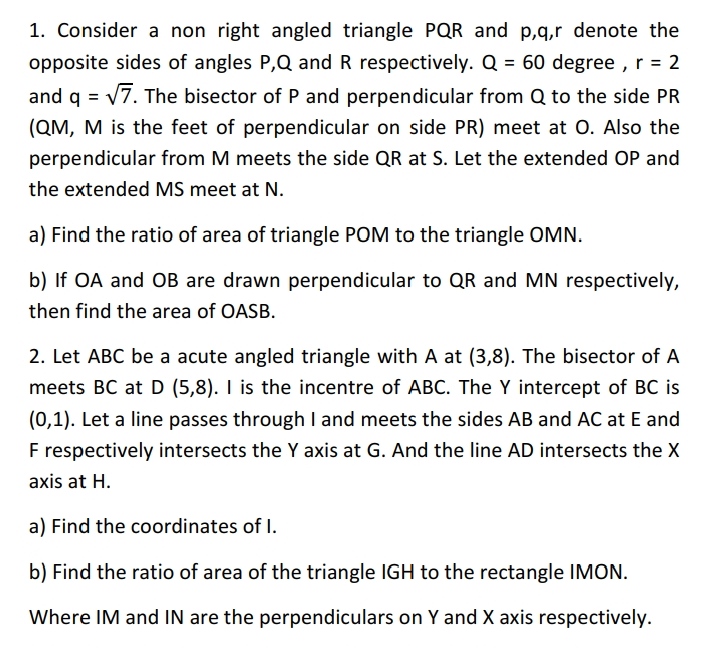
Mathematics Physics Chemistry Videos Login/Register Ask Question

32 - Trigonometry Questions Answers

Mathematics >> Trigonometry >> Properties of triangles IIT JEE
Asked By: TONY
is this question helpfull: 0 0 submit your answer
Mathematics >> Trigonometry >> Properties of triangles IIT JEE
Asked By: RAM KUMAR
is this question helpfull: 0 0 submit your answer
Mathematics >> Trigonometry >> De Moivres Theorem IIT JEE
Are these formulaes valid?
Asked By: LUFFY
is this question helpfull: 0 0 submit your answer
Joshi sir comment
Mathematics >> Trigonometry >> Trigonometrical Equations AIEEE
Asked By: UTTAM RAJ
is this question helpfull: 1 0 submit your answer
Joshi sir comment
Maths >> Trigonometry >> Complex Numbers Engineering Exam

what is the angular difference between the +j and -j ?

OR 

+4j and -4j  ?

 

Asked By: ADARSH KUMAR
is this question helpfull: 7 1 submit your answer
Joshi sir comment

180 degree

Maths >> Trigonometry >> Inverse Trigonometry AIEEE

sin‾¹(sin2) + sin‾¹(sin4) + sin‾¹(sin6) = ?

Asked By: BONEY HAVELIWALA
is this question helpfull: 20 6 submit your answer
Joshi sir comment

sin‾¹(sin2) + sin‾¹(sin4) + sin‾¹(sin6)

= (π-2) + (π-4) + (6-2π) 

= 0

Maths >> Trigonometry >> General Trigonometry Board Exam

Solve

tanx-3cotx=2tan3x(0<x<360)

cosx-sinx=cosy-siny

cos2xcotx+1=cos2x+cotx

Asked By: YUMESH
is this question helpfull: 6 0 submit your answer
Joshi sir comment

tanx - 3/tanx = 2[3tanx-tan3x]/[1-3tan2x]

so [tan2x - 3]/tanx = 2tanx[3-tan2x]/[1-3tan2x]

so [tan2x - 3][1-3tan2x] = 2tan2x[3-tan2x]

so [tan2x - 3][1-3tan2x] + 2tan2x[tan2x-3] = 0

so [tan2x - 3][1-tan2x] = 0

so tanx = 1, -1, √3, -√3

now check the values satisfying last eq.

then put these values in 2nd eq. to get answer.

Maths >> Trigonometry >> General Trigonometry AIEEE

Find the value :-

(tan69 +tan66) / (1 - tan69.tan66)

Asked By: ADARSH
is this question helpfull: 4 1 read solutions ( 2 ) | submit your answer
Joshi sir comment

use formula of tan(A+B)

Maths >> Trigonometry >> General Trigonometry AIEEE

Find the max. and min. value of

y = 7cosA + 24sinA

Asked By: ADARSH
is this question helpfull: 1 0 read solutions ( 3 ) | submit your answer
Joshi sir comment

   7cosA + 24sinA

= 25[7cosA/25 + 24sinA/25] = 25[cosAcosB + sinAsinB]                          where tanB = 24/7

= 25cos[A-B]                    max and min of cos[A-B] = 1 and -1                

so max. = 25  and min. = -25

Maths >> Trigonometry >> General Trigonometry Engineering Exam

Prove that :-

tanA + 2tan2A + 4tan4A + 8cot8A = cosecA / secA

Asked By: ADARSH
is this question helpfull: 0 0 submit your answer
Joshi sir comment

We have to prove

tanA + 2tan2A + 4tan4A + 8cot8A = cosecA / secA

or tanA + 2tan2A + 4tan4A + 8cot8A = cotA

or 2tan2A + 4tan4A + 8cot8A = cotA - tanA

or tan2A + 2tan4A + 4cot8A = [1-tan2A]/2tanA

or tan2A + 2tan4A + 4cot8A = cot2A

or 2tan4A + 4cot8A = cot2A - tan2A

or tan4A + 2cot8A = [1-tan22A]/2tan2A

or tan4A + 2cot8A = cot4A

or 2cot8A = cot4A-tan4A

or cot8A = [1-tan24A]/2tan4A

it is true so proved

Login Here

Register