303 - Mechanics part 1 Questions Answers
A block of mass m takes t to slide down on a smooth inclined plane of angle of inclination and height h. If same block slids down on a rough inclined plane of same angle of inclination and some height and takes time n times of initial value, then coefficient friction between block and inclined plane is
the answer is [1- 1/n² ] tanθ
in first case acceleration is gsinθ
and in second case acceleration is gsinθ-μgcosθ
now use s = 1/2 a t2 in both the case and solve
A block of mass m is at rest on a rough inclined plane of angle of inclination θ . If coefficient of friction between the block and the inclined plane is required to move the block on the plane is
how the answer is mg[sinθ - μ cosθ] .
i think one line is missing in the question.
a small body starts sliding from a height h down an inclined groove passing into a half circle of radius h/2. find the speed of the bodywhen it reaches the highest point of its trajectory
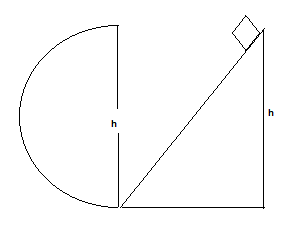
since body starts from height h at rest and ends in the same height so it will again be in rest
A BLOCK OF WOOD IS KEPT ON THE FLOOR OF A STATIONARY ELEVATOR. D ELEVATOR BEGINS TO DESCEND WITH AN ACCLN 12m/s2 . IF g =10 , THEN DISPLACEMENT OF BLOCK W.R.T. GROUND DURING FIRST 0.2 SEC. AFTER D START WILL BE ??
ANS 0.2m
s = ut + 1/2 at2
so s = 0 + 1/2 10(0.2)2
so s = 5(0.04) = 0.2
since elevator is moving with an acc. greater than g so we should take it a free fall
A body of mass 32kg is suspended by a spring balance from the roof of a vertically operating lift going downward from rest . At that instant the lift covered 20m and 50m , the spring balance showed 30 kg & 36 kg respt. the vel. is increasing at 20m & decreasing at 50m. Plzz sir explain this.
for downward journey
first case
32g-30g = 2g = 32a so a = g/16
second case
32g-36g = -4g = 32a so a = -g/8
so it is acceleration upto 20 m and retardation after that
d benches of a gallery in a cricket stadium r 1m wide & 1m high . a boy strikes d ball at a level of 1m above d ground & hits a sixer. d ball starts at 35m/s at an < of 530 with d horizontal . d benches r perpendicular to plane of motion & d first bench is 110m from d boy. ON WHICH BENCH WILL THE BALL HIT??
ANS 6TH
we know that y = xtanθ - gx2/2u2cos2θ
according to the given conditions
x = 110+n
y = n here n is the number of bench in which ball strikes
θ, u and g are given
A 6 kg bomb at rest explodes into three equal pieces P, Q and R. If P flies with speed 30 m/s and Q with speed 40 m/s making an angle 90° with the direction of P. The angle between the direction of motion of P and R is about
1. 143° 2. 127° 3. 120°

since initially bomb is in rest so initially momentum of the bomb = 0i+0j+0k
after explosion total momentum = 30mi+40mj+mv here v is a vector
now by momentum conservation 0 = 30mi+40mj+mv so v = -30i-40j
so vector along P is i and along R is -3i-4j
so by A.B = |A||B|cosθ
we get i.[-3i-4j] = 1*5*cosθ
so -3/5 = cosθ so θ = 127
If n balls hit elastically and normally on a surface per unit time and all the balls of mass m are moving with same velocity u, then force on surface is
2 mun . How ?
momentum change in elastic collision = mu-(-mu) = 2mu here after collision partical will retrace its direction
for n balls it will be 2mun
so force = rate of change of momentum = 2mun/1 = 2mun
A man of mass 50 kg carries a bag of weight 40 N on his shoulder. The force with which the floor pushes up his feet will be
1. 882 N 2. 530 N 3. 90 N 4. 600 N
reaction by ground is due to both bag and man so 50g+40 = 50*9.8+40 = 490+40 = 530 N
When a 4 kg rifle is fired, the 10 g bullet receives an acceleration of 3 x 10^6 cm/s^2 . The magnitude of the force acting on the rifle (in newton) is
1. 300 2. 200 3. 3000
force on the bullet = [10/1000]*3*104 = 300 N
so that will be same for rifle
