303 - Mechanics part 1 Questions Answers
Updated:
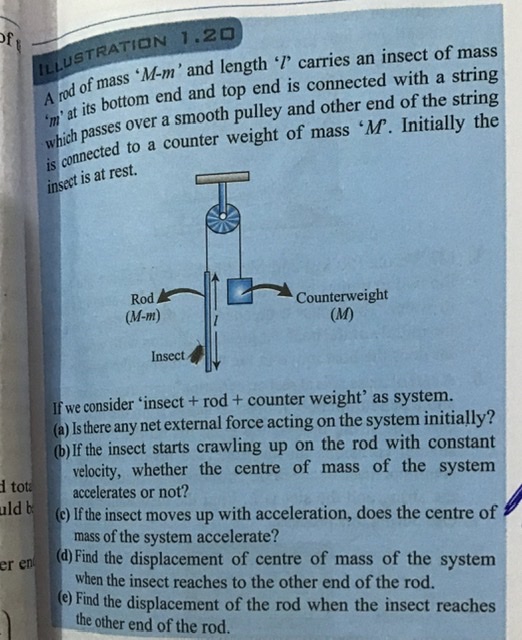
Initially there is no external force. Centre of mass of the system accelerates when insect moves with acceleration.
Now suppose displacement of insect at t = t is x with respect to rod, and y is the acceleration of rod in downward direction with respect to ground. Thus acceleration of counterweight is y with respect to ground. Now→xCM=[m(x-y)ˆj-(M-m)yˆj+Myˆj]/2MNow solve for x = l,
For dynamics, take friction upward in free body diagram of insect and downward in the free body diagram of rod.

Now solve
Inform for any issue.
A cylindrical pipe of radius r is rolling towards a frog sitting on the horizontal ground. Center of the pipe is moving with velocity v. To save itself ,the frog jumps off and passes over the pipe touching it only at top. Denoting air time of frog by T, horizontal range of the jump by R and acceleration due to gravity by g. Find T and R.
According to given condition 2r = uy2/2g,T= 2uy/g =2√4gr/g=4√r/gAt top point due to touch, vecocity of the frog be ux−2v.R=[uxuy/g]+[ux−2v)uy/g]=[uy/g](2ux−2v)=[2uy/g](ux−v) =T(ux−v)Now minimum possible value of velocity of frog at top to cross the cylinder is √gr (By the concept of vertical circle)now solve carefully without error
A particle of mass m = 2kg is placed on the top of a hemisphere of M 4 kg. The hemisphere is placed on smooth ground. The particle is displaced gently. Then ratio of normal and pseudo force(seen from hemisphere frame) acting on particle when theta = 30 degree. Assume particle remain in contact with hemisphere.

Let the acceleration of hemisphere is ahN sinθ = Mah⇒N/mah = M/msinθ Here pseudo force is considered due to acceleration of hemisphere
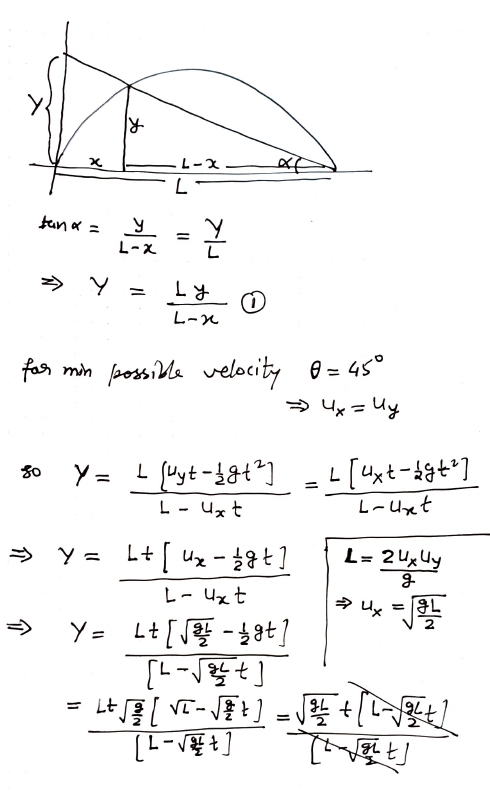
A small ball is thrown from foot of a wall with minimum possible velocity to hit a bulb B on the ground at a distance L away from the wall.Find the expression for height h of shadow of the ball on the wall as a function of time t.Acceleration due to gravity is g.

In first case F = 2T = 150so T = 75 newton, Now in second case system is free to moveacceleration of the system a = F/20for 10 kg rod F-2T = 10a = 10(F/20) = F/2⇒F-(F/2)=2T ⇒F=4T = 300 newton
what is the moment of inertia of a cube about its diagonal?
Moment of inertia of a cube about a line perpendicular toits one face and passing through its centre is ma2/6so about diagonal inertia = l2Ix+m2Iy+n2Iz = (l2+m2+n2)Ix (here Ix=Iy=Iz)= Ix = ma2/6l,m,n are direction cosines of three lines passing througecentre of cube and perpendicular to each other
While making this paper, I stayed up till late at night working with candle light. I had two
candles of equal length L lit and placed at the same time, as shown in the figure. Immediately
thereafter I noticed that the shadow of the first candle on the left wall is not changing in
length, and the shadow of the second candle on the right wall is being shortened at a speed v.
(Take d1
= 10 cm, d2
= 20 cm, d3
= 30 cm, v = 1 mm/s, L = 20 cm.)
Problem is interesting yet not complete. Complete it to get answer
thanks
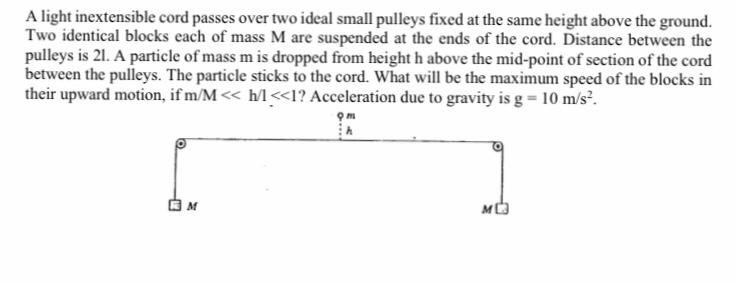
At a general point-2Tsinθ = ma (θ is measured from horizontal)⇒a = -2Mgθ/m = -2Mgy/mlcompare with a = -ω2yω=√2Mgml (1)Now for max displacement of small mmg(h+y) = 2Mgx and x = y2/2lon solving this quadratic eq. for y/l we get yl-m2M=√mMhl⇒yl=√mMhl (given that mM <<hl)so x = m2Mh (2)so vmax = xωnow solve
Two boys enter a running escalator at the ground floor of a shopping mall . The first boy repeatedly follows a cycle of p1=1 step up and then q1=2 steps down whereas the second boy repeatedly follows a cycle of p2=2 steps up and then q2=1 step down. Both of them move relative to escalator with a speed v=50cm/s . If the boys take t1=250s and t2=50s respectively to reach the first floor in complete number of cycles, how fast is the escalator running ?
let u and v are the speeds of boy and escalatoraccording to given condition [1(u+v)T-2(u-v)T]n1=[2(u+v)T-1(u-v)T]n2 ⇒[1(u+v)-2(u-v)]Tn1=[2(u+v)-1(u-v)]Tn2Given that Tn1=250 and Tn2=50⇒[1(u+v)-2(u-v)]250=[2(u+v)-1(u-v)]50now solve it for v, u is given
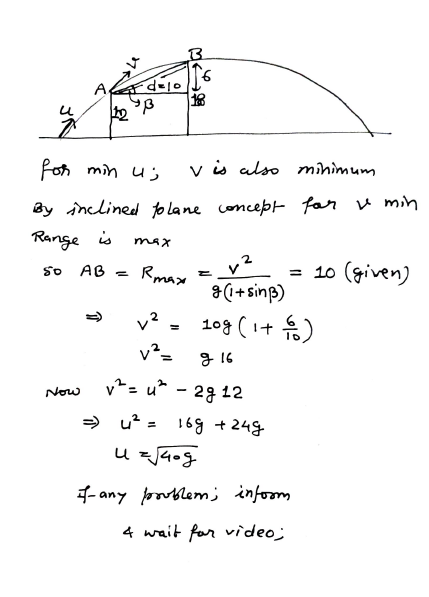
A particle projected from the ground passes two points , which are at height 12m and 18m above the ground and a distance d=10m apart . What could be the minimum speed of projection. Acceleration due to gravity is g=10m/s^2 .