576 - Physics Questions Answers

Current comes out from the surface of one sphere symmetrically
and enters to the surface of other symmetrically.
Resistance of the medium around one sphere is
∫∞rρdx4πx2=ρ4π[-1x]on putting limits resistance around one sphere =k1rsimilar for other sphere so net R1=2krIn second case radius of one sphere is half means r/2Thus R2 = kr+kr/2=3krnow for same battery i1R1=i2R2solve and wait for video
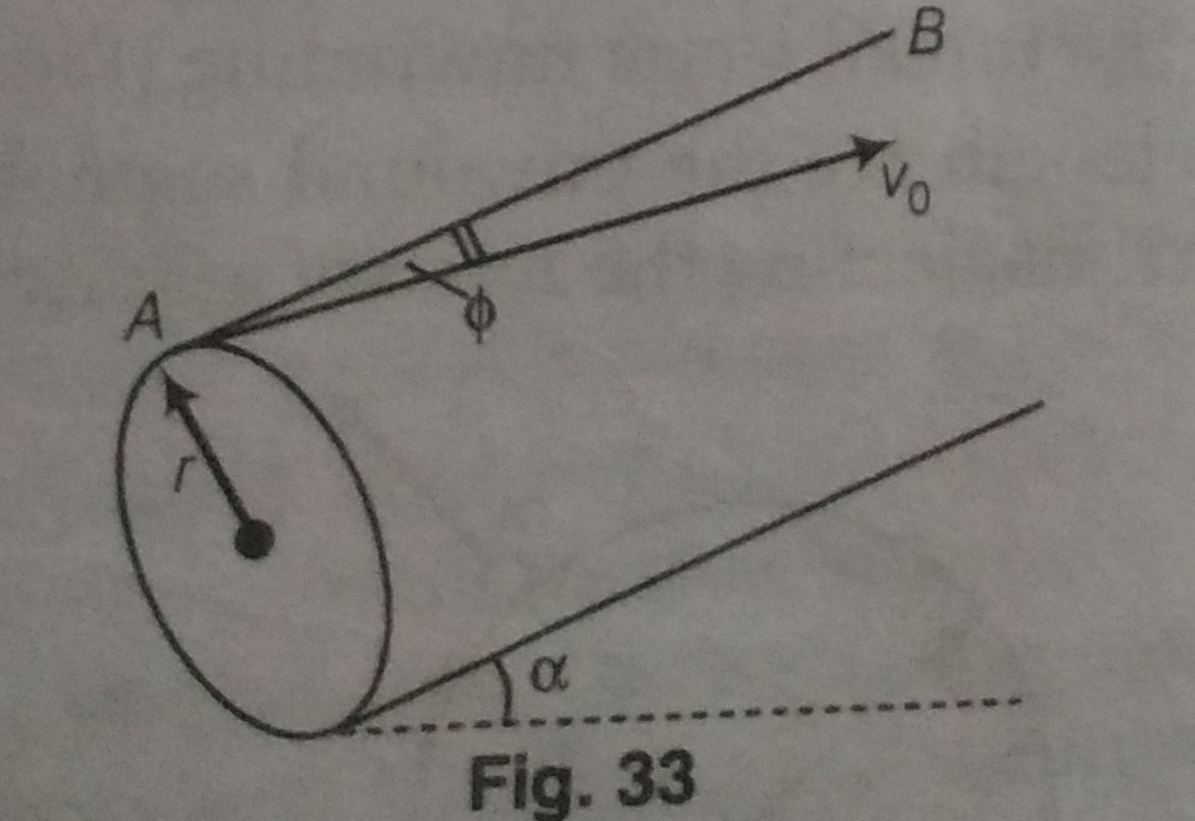
A long smooth cylindrical pipe of radius r is tilted at an angle alpha to the horizontal. A small body at point A is pushed upwards along the inner surface of the pipe so that the direction of its initial velocity forms an angle phi with generatrix AB. Determine the minimum initial velocity V at which the body starts moving upwards without being separated from the surface of the pipe
At the front of the cylinder, see a tilted circle Now compare this problem with vertical circular motion.A is the top point of that path. We know that the minimum velocity at top point tangential to path of vertical circle should be √gr.Here due to tilt of this circle effective gravity = gcosαso vsinφ =√gcosαrnow solveinform for any issuewait for video

According to first given condition velocity component of rain = u
let vertical component of rain = v
According to second condition
velocity of rain relative to man
=(nu-u)(-ˆi)+v(-ˆj)here direction of motion of man is ˆi and vertically upward direction is considered as ˆjNow draw triangle for this vector and get cotθsolve itinform for any issue
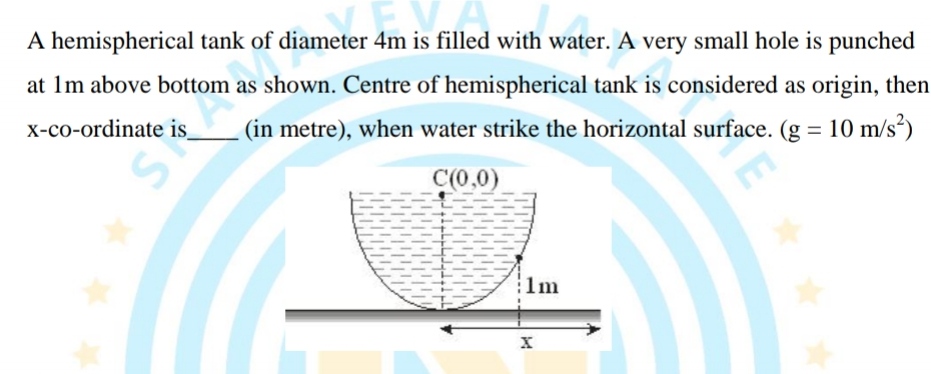
By Bernoullis theorem, velocity of water jet = √2ghWater jet comes out perpendicular to surface.
make its horizontal and vertical components
By vertical component, calculate time, then use it with
horizontal velocity component to get distance along x axis
Finally calculate distance from centre, along x axis
Inform for any confusion
Updated:
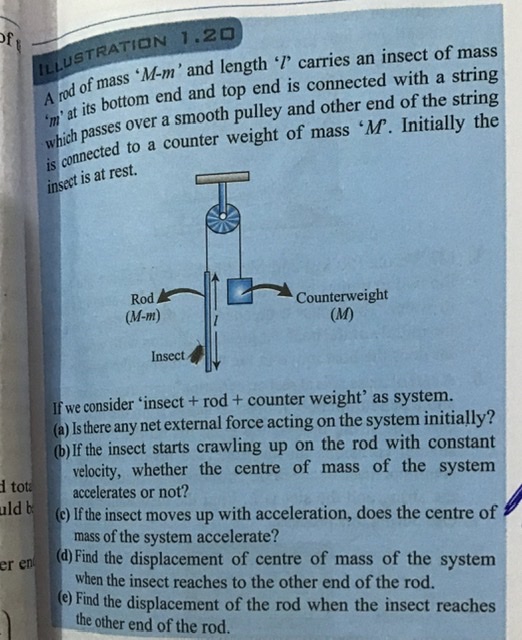
Initially there is no external force. Centre of mass of the system accelerates when insect moves with acceleration.
Now suppose displacement of insect at t = t is x with respect to rod, and y is the acceleration of rod in downward direction with respect to ground. Thus acceleration of counterweight is y with respect to ground. Now→xCM=[m(x-y)ˆj-(M-m)yˆj+Myˆj]/2MNow solve for x = l,
For dynamics, take friction upward in free body diagram of insect and downward in the free body diagram of rod.

Now solve
Inform for any issue.

Consider an electron trapped in an infinite well whose width is 98.5 pm. If it is in a state with n = 15, what are (i) its energy? (ii) the uncertainty in its momentum? (iii) the uncertainty in its position?
Let width=bFor nth state nλ2=b ⇒λ=2bnNow E =p22m = (hλ)22mSolve after putting the value of λ Uncertainty in position=bNow use ∆p.∆x = nh/2π for finding uncertainty in momentum
A solid conducting sphere of radius R is placed in a uniform electric field E as shown in figure-1.441. Due to electric field non uniform surface charges are induced on the surface of the sphere. Consider a point A on the surface of sphere at a polar angle θ from the direction of electric field as shown in figure. Find the surface density of induced charges at point A in terms of electric field and polar angle θ.
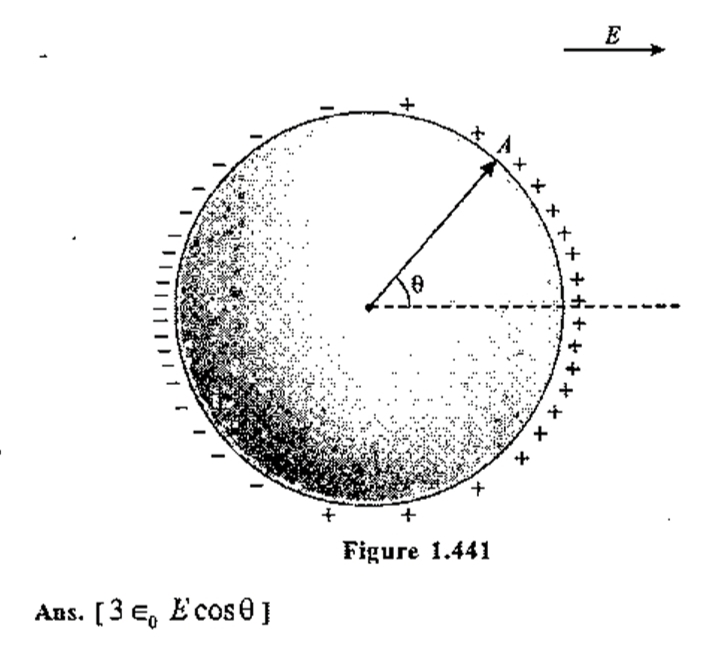
To nullify the electric field inside, the conducting sphere creates a situation in which electric field inside due to charge on sphere would be same as that of given electric field. Now this is the case, which is same as Irodov 3.17.
See the video now
link:
FinallyE = σ03ε0 = σ3ε0cosθNow solve

By superposition principle, it is solved in minimum time and effort, other method is very complex. So use superposition principle.
Divide current in point 1 and 2, considering symmetry throughout, we get the equivalent resistance of the whole network except resistor R between 1 and 2 is 2R
Now 2R and R in parallel between 1 and 2
Now solve
A cylindrical pipe of radius r is rolling towards a frog sitting on the horizontal ground. Center of the pipe is moving with velocity v. To save itself ,the frog jumps off and passes over the pipe touching it only at top. Denoting air time of frog by T, horizontal range of the jump by R and acceleration due to gravity by g. Find T and R.
According to given condition 2r = uy2/2g,T= 2uy/g =2√4gr/g=4√r/gAt top point due to touch, vecocity of the frog be ux−2v.R=[uxuy/g]+[ux−2v)uy/g]=[uy/g](2ux−2v)=[2uy/g](ux−v) =T(ux−v)Now minimum possible value of velocity of frog at top to cross the cylinder is √gr (By the concept of vertical circle)now solve carefully without error
